How to choose the diameter of a heating pipe
It will not be possible to calculate exactly what cross-section of pipes you need.
You will have to choose from several options. And all because the same effect can be achieved in different ways. Let's explain
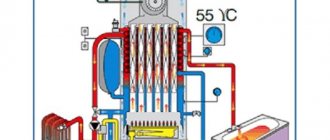
It is important for us to deliver the right amount of heat to the radiators and at the same time achieve uniform heating of the radiators. In systems with forced circulation we do this using pipes, coolant and a pump
In principle, all we need is to “drive” a certain amount of coolant over a certain period of time. There are two options: install pipes of a smaller diameter and supply coolant at a higher speed, or make a system of a larger cross-section, but with less traffic. Usually the first option is chosen. And that's why:
- the cost of products with a smaller diameter is lower;
- they are easier to work with;
- when laid open, they do not attract so much attention, and when laid in the floor or walls, smaller grooves are required;
- with a small diameter, there is less coolant in the system, which reduces its inertia and leads to fuel savings.
Since there is a certain set of diameters and a certain amount of heat that needs to be delivered through them, it is unreasonable to calculate the same thing every time. Therefore, special tables have been developed, according to which, depending on the required amount of heat, the speed of movement of the coolant and the temperature indicators of the system, the possible size is determined. That is, to determine the cross-section of pipes in the heating system, find the required table and select the appropriate cross-section from it.
The diameter of the heating pipes was calculated using the following formula (you can calculate it if you wish). Then the calculated values were recorded in a table.
Formula for calculating the diameter of a heating pipe
Where:
D - required pipeline diameter, mm ∆t° - temperature delta (difference between supply and return), °C Q - load on a given section of the system, kW - determined amount of heat required to heat the room V - coolant velocity, m/s - selected from a certain range.
In individual heating systems, the coolant movement speed can be from 0.2 m/s to 1.5 m/s. Based on operating experience, it is known that the optimal speed is in the range of 0.3 m/s - 0.7 m/s. If the coolant moves more slowly, air jams occur; if it moves faster, the noise level increases significantly. The optimal speed range is selected in the table. The tables are designed for different types of pipes: metal, polypropylene, metal-plastic, copper. The values are calculated for standard operating modes: high and medium temperatures. To make the selection process more clear, let’s look at specific examples.
What factors are taken into account when calculating water consumption?
Determining water flow by pipe diameter allows you to obtain data that is very close to real, but not always. In addition to the pipe diameter, the real flow rate is affected by a number of factors:
- pressure level. With higher pressure in the pipeline system, consumers will receive a larger volume of water. Calculating water flow by pipe diameter and pressure allows you to obtain more accurate data than using only one parameter. Based on these values, the required pipe wall thickness is determined;
- The water pressure in the system depends on changes in the diameter of the pipes, bends and turns, branches, and the presence of shut-off valves. The more complex the configuration of the water supply system, the more difficult it is to determine the real indicators of water flow through the pipe at the pressure specified in accordance with SNiP;
- the force of friction that impedes the movement of water flow; with a greater length of the system, the flow of water through the pipe is significantly reduced, since the speed of movement of the liquid decreases;
- roughness of the internal walls of the water pipe. Modern polymer structures have approximately ten percent higher throughput than the newest products made from traditional materials - concrete, cast iron and steel;
- During long-term operation, the internal surface of the pipeline becomes clogged with various deposits. Changes in the internal topography due to contamination can hardly be calculated using mathematical formulas. So, it will be impossible to accurately determine the amount of water passing through the pipe. New polymer materials make it possible not to take into account the factor of gradual blockage of the system, since the formation of growths on their inner surface is practically eliminated.
Water consumption will depend on the configuration of the water supply system, as well as the type of pipes from which the network is installed
So, when calculating water pressure depending on the diameter of the pipe, without taking into account other factors that affect the actual flow of liquid, significant errors can be made.
Calculation of diameter for a two-pipe heating system
We will calculate using the example of a simple two-story house. On each floor we have two wings. The house itself will have a two-pipe heating system with the following parameters:
- total heat loss – 36 kW;
- loss on the 1st floor – 20 kW;
- loss on the 2nd – 16 kW;
- polypropylene pipes were installed;
- system operation in 80/60 mode;
- temperature – 20 C.
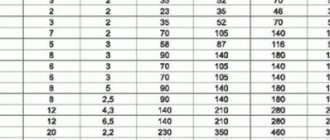
Below is table (a) based on the data from which we will determine the required pipe diameter. In the table, cells with the best (optimal) fluid velocity are marked in green.
Let's count. Through the section of pipe that connects the first fork and the boiler, the entire volume of liquid passes through, therefore, all the heat, and this is 38 kW. Let's determine which pipe we need to take here.
We take our table, look for the corresponding line in it, then go through the green cells and look up. What do we see? And we see that with such parameters two options suit us: 50 and 40 mm. Naturally (this was written about above), we choose a smaller diameter of the pipe for heating the house, 40 mm.
Next we look at the fork, which divides the coolant movement into the second and first floors (16 and 20 kW). Again we look at the values from the table and find that in both directions a pipe diameter of 32 mm is needed.
We have two wings on each floor. The circuit is also divided into two branches. We count the first floor:
20 kW / 2 = 10 kW per wing
Second floor by analogy:
16 kW / 2 = 8 kW per wing
Again, we take our table and determine that in these areas we need a pipe with a cross-section of 25 mm. It is also clearly seen from the table that we will use this diameter until the load drops to 5 kW, then we will use 20 mm pipes.
In this simple way, we calculated all the pipe diameters for heating the house of the polypropylene pipes we needed for a two-pipe heating system.
For the reverse supply of water, you do not need to calculate anything, everything is much simpler: you do all the wiring with pipes of the same diameter as for the direct supply. As you can see, there is nothing complicated. All you need is a good table suitable for a specific case.
Some nuances of calculating the diameter for metal pipes
But on extended systems, it may happen that the very last heating elements in the chain will be cold or slightly warm. This is also a consequence of incorrect choice of pipe diameter. Fortunately, heat loss can be easily calculated:
q = k * 3.14 * (tv-tp) q - heat loss per 1 meter (W/s); k – heat transfer coefficient (W * m/s); tв - temperature of hot supplied water (C); tп — ambient temperature (C).
Let's take a pipe with a diameter of 40 mm. Let's say the wall is 1.4 mm thick. Material – steel. Let's calculate:
q = 0.272 * 3.15 * ( 80 – 22 ) = 49 W/s
Here is another proof of why you need to take a pipe diameter for heating a house with a smaller diameter. After all, it is clear that the thicker the pipe, the much more heat we will lose.
And in this example, we received losses of almost 50 W per 1 meter of distance. And if the system is quite extended, then all the heat can be lost.
But don't be upset! Such accurate calculations are needed only for multi-storey residential buildings. For individual heating systems, everything is simpler: calculations are rounded up and this gives a certain margin.
How the calculation is made according to the installed boiler: the wiring depends on many factors
First, we determine which pipe is required in the section from the boiler to the first divergence in the house. Let's say the amount of heat is 38 kW. In the section corresponding to this indicator, we go to the cells colored in pink and look at what diameters of pipes for heating a private house correspond to these zones. We determine that these are pipes of 40 and 50 mm. We choose the smaller one, that is, 40 mm. The diameter of the pipe for the heating system is selected by a real specialist in this matter.
Next comes the branching of the pipeline in the house. For example, on two floors. The heat absorption is higher on the lower floor. Let's assume that 20 kW are absorbed by the first floor, and 18 are transferred to the second floor. From the table we determine that this heat transfer corresponds to a cross-sectional size of 32 mm.
At each floor, the pipeline is again divided into two branches. We get 10 kW on the first floor and 9 on the second. From the table we see that these parameters correspond to a 25 mm pipe. There are two wings on each floor. Again the heat value is divided by two and the result is 5 and 4.5 kW. This is followed by the division of the pipeline into rooms, and heat consumption reaches 5 kW. The diameter is reduced to 20 mm. But as practical experience shows, switching to “twenty” makes sense only when heat consumption is up to 3 kW. The return is performed in the same order.
The number of heating radiator sections is calculated based on the area of the room where the radiators are installed, and based on the passport data of the radiators themselves, which indicate the power of the sections.
In conclusion, it should be said that the heating of a country house does not work around the clock and regularly. It is turned on only when there are people in the house. And in winter there is a danger of freezing of the heating circuit, rupture of pipes and radiators. To prevent this from happening, non-freezing heating liquid is poured into the boiler. which, when mixed with water, lowers its freezing point, protects the steel components of the heating system from corrosion from the inside. By the way, polypropylene pipes are capable of expanding somewhat, which protects them from ruptures due to freezing, therefore, when organizing heating, preference is given to this type of pipeline. The diameter of the heating pipe must meet all the requirements of the system in general.
Two-pipe circuit in a private house
First, let's generalize a little. Let’s take, for example, calculating the diameter of polypropylene pipes for heating in a private house. Basically, products with a cross section of 25 mm are used for the circuit, and bends to radiators are set to 20 mm. Due to the fact that the size of the heating pipes in a private house, used as pipes to the batteries, is smaller, the following processes occur:
the coolant speed increases; circulation in the radiator improves; The battery warms up evenly, which is important when connected at the bottom.
Combinations of a main circuit diameter of 20 mm and branches of 16 mm are also possible.
To verify the above data, you can calculate the diameter of pipes for heating a private house yourself. This will require the following values:
Knowing the number of heated square meters, we can calculate the boiler power and what pipe diameter to choose for heating. The more powerful the heater, the larger the cross-section of the product that can be used in tandem with it. To heat one square meter of space, 0.1 kW of boiler power is required. The data is valid if the ceilings are standard 2.5 m;
The indicator depends on the region and wall insulation. The bottom line is that the greater the heat loss, the more powerful the heater should be. To get around the complex calculations that are not appropriate in the approximate calculation, simply add 20% to the boiler power calculated above;
water speed in the circuit.
Allowed coolant speed is in the range from 0.2 to 1.5 m/s. Moreover, in most calculations of the diameter of pipes for heating with forced circulation, it is customary to take an average value of 0.6 m/s. At this speed, noise from friction of the coolant against the walls is eliminated;
how much the coolant cools down.
To do this, subtract the return temperature from the supply temperature. Naturally, you cannot know the exact data, especially since you are at the design stage. Therefore, operate with average data, which are 80 and 60 degrees, respectively. Based on this, heat loss is 20 degrees.
Now the calculation itself is how to choose the diameter of the pipe for heating. To do this, let's take a formula that initially contains two constant values, the sum of which is 304.44.
Conditional flow of the circuit, squared = 304.44 x (area of the room x 0.1 kW + 20%) / heat loss of the coolant / flow rate.
The last step is to extract the square root of the result. For clarity, let’s calculate what pipe diameter to use for heating a private house with one floor with an area of 120 m2:
304.44 x (120 x 0.1 + 20%) / 20 / 0.6 = 368.328
Now let's calculate the square root of 368.328, which is equal to 19.11 mm. Before choosing the diameter of the heating pipe, we once again emphasize that this is the so-called conditional diameter. Products made from different materials have different wall thicknesses. For example, polypropylene has thicker walls than metal-plastic. Since we have knitted a polypropylene circuit as a sample, we will continue to consider this material. The marking of these products indicates the outer cross-section and wall thickness. Using the subtraction method, we find out the size we need and select it in the store.
The ratio of the outer and inner diameter of polypropylene pipes
For convenience, we will use the table.
Based on the results of the table, we can conclude:
- if a nominal pressure of 10 atmospheres is sufficient, then an outer section of the heating pipe of 25 mm is suitable;
- if a nominal pressure of 20 or 25 atmospheres is required, then 32 mm.
In this article I will tell you how to professionally calculate the diameter of a pipe. Useful formulas will be provided. You will find out what pipe diameter you need for water pipes. It is also very important not to confuse the calculation of the selection of pipe diameter for water supply with the calculation for heating. Since a low flow of water movement is sufficient for heating. The formula for calculating the diameter of pipes is radically different, since water supply requires high water flow rates.
How to calculate the diameter of a heating pipe is described here: Calculating the diameter of a heating pipe
As for the tables for calculating the diameter of the pipe, this will be discussed in other articles. I can only say that this article will help you find the diameter of pipes without tables, using special formulas. And the tables were invented simply to simplify the calculation process. In addition, in this article you will understand what the entire result of the required diameter consists of.
Watch the video:
Buy the program
To get a calculation of the diameter of a pipe for water supply, you need to have ready-made numbers:
Here are some formulas that will help you find the flow rate:
| S-Sectional area m2 π-3.14-constant - the ratio of the circumference of a circle to its diameter. r-Radius of a circle equal to half the diameter Q-water flow m3/s D-Inner diameter of the pipe |
0.25l/s=0.00025m3/s
12mm=0.012m
V=(4*Q)/(π*D2)=(4*0.00025)/π*0.0122=2.212 m/s
Next, we find the Reynolds number using the formula:
Read more about the Reynolds number in the article: Modes of fluid motion (laminar and turbulent)
Re=(V*D)/ν=(2.212*0.012)/0.00000116=22882
ν=1.16*10-6=0.00000116. Taken from the table. For water at a temperature of 16°C.
Δe=0.005mm=0.000005m. Taken from the table for a metal-plastic pipe.
Next, we check the table where we find the formula for finding the coefficient of hydraulic friction.
I fall into the first area and I accept the Blasius formula for calculation.
λ=0.3164/Re0.25=0.3164/228820.25=0.0257
Next, we use the formula to find pressure loss:
| h-pressure loss, here it is measured in meters. λ-coefficient of hydraulic friction. L-length of the pipeline is measured in meters. D is the internal diameter of the pipe, that is, the diameter of the fluid flow. Must be inserted into the formula in meters. V is the velocity of fluid flow. Measured [Meter/second]. g-gravitational acceleration is 9.81 m/s2 |
h=λ*(L*V2)/(D*2*g)=0.0257*(10*2.2122)/(0.012*2*9.81)=5.341 m.
And so: At the inlet we have 2 atmospheres, which is equal to 20 meters of pressure.
If the result obtained is 5.341 meters less than the input pressure, then the result satisfies us and the pipe diameter with an internal diameter of 12 mm is suitable!
If not, then it is necessary to increase the diameter of the pipe.
But keep in mind that if you take into account the pipe that goes from the basement through the risers to your fifth floor, the result may not be satisfactory. And if your neighbors take away the flow of water, then the input pressure may decrease accordingly. So keeping in mind a reserve of two or three times is already good. In our case, the margin is four times larger.
Let's try this for the sake of experiment.
We have 10 meters in the pipe along the way, there are four angles (elbows). These are hydraulic resistances and are called local hydraulic resistances. For a 90 degree elbow there is a calculation formula:
Read more about this in the section: Local hydraulic resistance.
| h-pressure loss, here it is measured in meters. ζ is the resistance coefficient. For a knee it is approximately one if the diameter is less than 30mm. V is the velocity of fluid flow. Measured [Meter/second]. g-gravitational acceleration is 9.81 m/s2 |
h=ζ*(V2)/2*9.81=0.249 m.
Since we have 4 squares, we multiply the result by 4 and get 0.996 m. Almost another meter.
Task 2:
The steel (iron) pipe is laid 376 meters long with an internal diameter of 100 mm; there are 21 bends along the length of the pipe (angular turns 90°C). The pipe is laid with a difference of 17 m. That is, the pipe goes up relative to the horizon to a height of 17 meters. Pump characteristics: Maximum head 50 meters (0.5 MPa), maximum flow 90 m3/h. Water temperature 16°C. Find the maximum possible flow rate at the end of the pipe.
Given:
| D=100 mm = 0.1m L=376m Geometric height=17m Bends 21 pcs Pump pressure=0.5 MPa (50 meters of water column) Maximum flow=90m3/h Water temperature 16°C. Steel iron pipe |
Find the maximum flow rate =?
Solution:
To solve, you need to know the pump schedule: Dependence of flow on pressure.
I chose a visually similar graph of all pumps; it may differ from the real one by 10-20%. For a more accurate calculation, you need a pump schedule, which is indicated in the pump passport.
In our case, the graph will look like this:
Look, I marked 17 meters with a broken line along the horizon and at the intersection along the curve I get the maximum possible flow rate: Qmax.
According to the graph, I can safely say that due to the difference in height, we lose approximately: 14 m3/hour. (90-Qmax=14 m3/h).
There is no direct formula that gives a direct calculation of finding the flow rate, and if it does exist, then it has a stepwise nature and some logic that can confuse you - completely. The stepwise calculation is obtained because in the formula there is a quadratic feature of pressure loss in dynamics (motion).
Therefore, we solve the problem step by step.
Since we have a flow range from 0 to 76 m3/hour, I would like to check the pressure loss at a flow rate of 45 m3/hour.
Finding the speed of water movement
Q=45 m3/h = 0.0125 m3/sec.
V = (4•0.0125)/(3.14•0.1•0.1)=1.59 m/s
Finding the Reynolds number
ν=1.16•10-6=0.00000116. Taken from the table. For water at a temperature of 16°C.
Re=(V•D)/ν=(1.59•0.1)/0.00000116=137069
Δe=0.1mm=0.0001m. Taken from the table for a steel (iron) pipe.
Next, we check the table, where we find the formula for finding the coefficient of hydraulic friction.
I get it in the second area, provided
10•D/Δe
10•0,1/0,0001
10 000
λ=0.11(Δe/D + 68/Re)0.25=0.11•(0.0001/0.1 + 68/137069)0.25=0.0216
Next we finish with the formula:
h=λ•(L•V2)/(D•2•g)= 0.0216•(376•1.59•1.59)/(0.1•2•9.81)=10.46 m .
As you can see, the loss is 10 meters. Next, we determine Q1, see the graph:
Now we make the original calculation at a flow rate of 64 m3/hour
Q=64 m3/h = 0.018 m3/sec.
V = (4•0.018)/(3.14•0.1•0.1)=2.29 m/s
Re=(V•D)/ν=(2.29•0.1)/0.00000116=197414
λ=0.11(Δe/D + 68/Re)0.25=0.11•(0.0001/0.1 + 68/197414)0.25=0.021
h=λ•(L•V2)/(D•2•g)= 0.021•(376•2.29 •2.29)/(0.1•2•9.81)=21.1 m.
We mark on the graph:
Qmax is at the intersection of the curve between Q1 and Q2 (Exactly the middle of the curve).
Answer: The maximum flow rate is 54 m3/h. But we solved this without resistance when cornering.
To check, let's check:
Q=54 m3/h = 0.015 m3/sec.
V = (4•0.015)/(3.14•0.1•0.1)=1.91 m/s
Re=(V•D)/ν=(1.91•0.1)/0.00000116=164655
λ=0.11(Δe/D + 68/Re)0.25=0.11•(0.0001/0.1 + 68/164655)0.25=0.0213
h=λ•(L•V2)/(D•2•g)= 0.0213•(376•1.91•1.91)/(0.1•2•9.81)=14.89 m .
Result: We got to Npot=14.89=15m.
Now let’s calculate the cornering resistance:
Formula for finding pressure on local hydraulic resistance:
Read more about this in the section: Local hydraulic resistance
| h-pressure loss here it is measured in meters. ζ is the resistance coefficient. For a knee it is approximately one if the diameter is less than 30mm. V is the velocity of fluid flow. Measured [Meter/second]. g-gravitational acceleration is 9.81 m/s2 |
ζ is the resistance coefficient. For a knee it is approximately one if the diameter is less than 30mm. For larger diameters it decreases. This is due to the fact that the influence of the speed of water movement relative to the turn is reduced.
I looked in different books on local resistances for turning pipes and bends. And he often came to the calculation that one strong sharp turn is equal to a coefficient of one. A sharp turn is considered if the radius of the turn does not exceed the diameter. If the radius exceeds the diameter by 2-3 times, then the coefficient value decreases significantly.
Read more about this in the section: Local hydraulic resistance
Let's take ζ = 1.
Speed 1.91 m/s
h=ζ•(V2)/2•9.81=(1•1.912)/( 2•9.81)=0.18 m.
We multiply this value by the number of bends and get 0.18•21=3.78 m.
Answer: at a speed of 1.91 m/s, we obtain a pressure loss of 3.78 meters.
Let's now solve the whole problem with taps.
At a flow rate of 45 m3/hour, we obtained a pressure loss along the length: 10.46 m. See above.
At this speed (2.29 m/s) we find the cornering resistance:
h=ζ•(V2)/2•9.81=(1•2.292)/(2•9.81)=0.27 m. multiply by 21 = 5.67 m.
We add up the pressure loss: 10.46 + 5.67 = 16.13 m.
We mark on the graph:
We solve the same thing only for a flow rate of 55 m3/h
Q=55 m3/h = 0.015 m3/sec.
V = (4•0.015)/(3.14•0.1•0.1)=1.91 m/s
Re=(V*D)/ν=(1.91 •0.1)/0.00000116=164655
λ=0.11(Δe/D + 68/Re)0.25=0.11•(0.0001/0.1 + 68/164655)0.25=0.0213
h=λ•(L•V2)/(D•2•g)= 0.0213•(376•1.91•1.91)/(0.1•2•9.81)=14.89 m .
h=ζ•(V2)/2•9.81=(1•1.912)/( 2•9.81)=0.18 m. multiply by 21 = 3.78 m.
Add up the losses: 14.89+3.78=18.67 m
We draw on the graph:
Answer:
Maximum flow=52 m3/hour. Without branches Qmax=54 m3/hour.
Now I think you understand how resistance to flow occurs. If it is not clear, then I am ready to hear your comments on this article. Write comments.
To avoid doing all the math manually, I prepared a special program:
Next article: How to select a pump according to technical parameters
| Like |
| Share |
| Comments (+) [ Read / Add ] |
All about the country house Water supply Training course. Automatic water supply with your own hands. For Dummies. Malfunctions of the well automatic water supply system. Water wells Well repair? Find out if you need it! Where to drill a well - outside or inside? In what cases does cleaning a well make no sense Why pumps get stuck in wells and how to prevent it Laying a pipeline from a well to a house 100% Protecting the pump from dry running Heating Training course. Do-it-yourself water heated floor. For Dummies. Warm water floor under laminate Educational Video course: According to HYDRAULIC AND THERMAL CALCULATIONS Water heating Types of heating Heating systems Heating equipment, heating batteries Heated floor system Personal article on heated floors Operating principle and operating diagram of a heated water floor Design and installation of a heated floor Do-it-yourself water heated floor Basics materials for heated water floors Installation technology of water heated floors System of heated floors Laying step and methods of laying heated floors Types of water heated floors All about coolants Antifreeze or water? Types of coolants (antifreeze for heating) Antifreeze for heating How to properly dilute antifreeze for the heating system? Detection and consequences of coolant leaks How to choose the right heating boiler Heat pump Features of a heat pump Heat pump operating principle About heating radiators Methods of connecting radiators. Properties and parameters. How to calculate the number of radiator sections? Calculation of thermal power and number of radiators Types of radiators and their features Autonomous water supply Scheme of autonomous water supply Construction of a well Cleaning a well with your own hands Experience of a plumber Connecting a washing machine Useful materials Water pressure reducer Hydraulic accumulator. Operating principle, purpose and configuration. Automatic air release valve Balancing valve Bypass valve Three-way valve Three-way valve with ESBE servo drive Thermostat for radiator Manifold servo drive. Selection and connection rules. Types of water filters. How to choose a water filter for water. Reverse osmosis Sludge filter Non-return valve Safety valve Mixing unit. Principle of operation. Purpose and calculations. Calculation of the CombiMix Gidrostrelka mixing unit. Operating principle, purpose and calculations. Indirect heating storage boiler. Principle of operation. Calculation of a plate heat exchanger Recommendations for the selection of PHE when designing heat supply facilities About contamination of heat exchangers Indirect water heating water heater Magnetic filter - protection against scale Infrared heaters Radiators. Properties and types of heating devices. Types of pipes and their properties Indispensable tools for a plumber Interesting stories A scary tale about a black installer Water purification technologies How to choose a filter for water purification Let's think about sewerage Sewage treatment plants in a rural house Tips for a plumber How to evaluate the quality of your heating and plumbing system? Professional recommendations How to choose a pump for a well How to properly equip a well Water supply for the garden How to choose a water heater An example of installing equipment for a well Recommendations for the configuration and installation of submersible pumps What type of water supply accumulator should you choose? Water cycle in an apartment - vent pipe Removing air from a heating system Hydraulics and heating engineering Introduction What is hydraulic calculation? Physical properties of liquids Hydrostatic pressure Let's talk about resistance to the passage of liquid in pipes Modes of liquid movement (laminar and turbulent) Hydraulic calculation of pressure loss or how to calculate pressure loss in a pipe Local hydraulic resistance Professional calculation of pipe diameter using formulas for water supply How to select a pump according to technical parameters Professional calculation of water heating systems. Calculation of heat loss of the water circuit. Hydraulic losses in a corrugated pipe Heat engineering. Author's speech. Introduction Heat transfer processes Thermal conductivity of materials and heat loss through the wall How do we lose heat with ordinary air? Laws of thermal radiation. Radiant warmth. Laws of thermal radiation. Page 2. Heat loss through a window Factors of heat loss at home Start your own business in the field of water supply and heating systems Question on hydraulic calculations Designer of water heating Pipe diameter, flow speed and coolant flow. We calculate the diameter of the heating pipe. Calculation of heat losses through the radiator. Power of the heating radiator. Calculation of the power of radiators. Standards EN 442 and DIN 4704 Calculation of heat loss through building envelopes Find heat loss through the attic and find out the temperature in the attic Selecting a circulation pump for heating Transfer of thermal energy through pipes Calculation of hydraulic resistance in a heating system Distribution of flow and heat through pipes. Absolute schemes. Calculation of a complex associated heating system Heating calculation. Popular myth Calculation of heating of one branch along the length and KMS Calculation of heating. Selection of pump and diameters Heating calculation. Two-pipe dead-end Heating calculation. Single-pipe sequential heating calculation. Two-pipe associated Calculation of natural circulation. Gravity pressure Calculation of water hammer How much heat is generated by pipes? We are assembling a boiler room from A to Z... Heating system calculation Online calculator Calculation program Heat loss of the room Hydraulic calculation of pipelines History and capabilities of the program - introduction How to make a calculation of one branch in the program Calculation of the KMS angle of the outlet Calculation of the KMS of heating and water supply systems Pipeline branching - calculation How to calculate in the program single-pipe heating system How to calculate a two-pipe heating system in a program How to calculate radiator flow in a heating system in a program Recalculation of radiator power How to calculate a two-pipe associated heating system in a program. Tichelman loop Calculation of a hydraulic separator (hydraulic arrow) in the program Calculation of a combined circuit of heating and water supply systems Calculation of heat loss through enclosing structures Hydraulic losses in a corrugated pipe Hydraulic calculation in three-dimensional space Interface and control in the program Three laws/factors for selecting diameters and pumps Calculation of water supply with self-priming pump Calculation of diameters from the central water supply Calculation of water supply for a private house Calculation of a hydraulic arrow and collector Calculation of a hydraulic arrow with many connections Calculation of two boilers in a heating system Calculation of a single-pipe heating system Calculation of a two-pipe heating system Calculation of a Tichelman loop Calculation of a two-pipe radial distribution Calculation of a two-pipe vertical heating system Calculation of a single-pipe vertical heating system Calculation of a warm water floor and mixing units Recirculation of hot water supply Balancing adjustment of radiators Calculation of heating with natural circulation Radial distribution of a heating system Tichelman loop - two-pipe associated Hydraulic calculation of two boilers with a hydraulic arrow Heating system (not Standard) - Another piping scheme Hydraulic calculation of multi-pipe hydraulic arrows Radiator mixed system heating - passing from dead ends Thermoregulation of heating systems Pipeline branching - calculation Hydraulic calculation for pipeline branching Calculation of a pump for water supply Calculation of warm water floor contours Hydraulic calculation of heating. Single-pipe system Hydraulic heating calculation. Two-pipe dead-end Budget option for a single-pipe heating system for a private house Calculation of the throttle washer What is KMS? Calculation of a gravity heating system Designer of technical problems Extension of a pipe Requirements SNiP GOSTs Requirements for a boiler room Question for a plumber Useful links for a plumber - Plumber - ANSWERS!!! Housing and communal problems Installation work: Projects, diagrams, drawings, photos, descriptions. If you're tired of reading, you can watch a useful video collection on water supply and heating systems
Selecting the diameter of heating pipes
It is impossible to accurately calculate what diameter of pipe to use for heating. You have to choose from several options for products on sale. The fact is that you can achieve the same result in different ways.
Let's say that it is necessary to deliver a certain amount of heat to the batteries and at the same time ensure uniform heating of the radiators. In heat supply structures with forced circulation of liquid, this is done using pumping equipment, coolant and pipes. In a word, it is necessary to ensure that a certain volume of the working environment is transported in a specific time.
This can be accomplished in one of two ways:
- lay pipes of a smaller cross-section and drive the liquid at a higher speed;
- equip a system with a large diameter, but with less intensity of movement.
As practice shows, property owners usually choose the first option for a number of reasons:
- Pipe products with a smaller diameter are cheaper and easier to install.
- With the open method of laying pipes, they are less noticeable, and when installed in the floor or walls, smaller grooves are needed.
- In the heat supply system, with a small diameter heating pipe in the apartment, there is a smaller volume of coolant, which reduces its inertia and saves fuel resources.
Since manufacturers produce pipe products of several diameters, through which a certain amount of heat can be delivered, it is not practical to calculate the same thing every time.
For this purpose, experts at one time developed tables. With their help, depending on the specific volume of heat, the speed of movement of the working medium and the temperature regime of the system, you can find out the possible diameter.
To determine this parameter for a heat supply structure, you should use the necessary table and use it to calculate which section of heating pipes to give preference to.
The calculation for it was carried out based on the formula, and then the results obtained were entered into the table:
D= , where:
D is the required diameter in millimeters;
∆t° – difference between the temperature values on the supply and return pipes, °C;
Q is the load on a section of the system, or rather the amount of heat required to heat a specific room, kW;
V is the speed of movement of the coolant, m/s.
In autonomous heat supply systems, the speed of movement of the working medium is in the range from 0.2 m/s to 1.5 m/s. As practice shows, the optimal value of this value is 0.3 m/s - 0.7 m/s.
If the coolant moves more slowly, air pockets form in the system, and if it moves faster, the noise level increases significantly. The optimal speed range is reflected in special tables. They were compiled separately for each type of pipe.
They were calculated for the operation of systems in standard modes, namely under conditions of medium and high temperatures. To make the process of determining which pipe diameter to choose for heating clear, it will be shown with specific examples.
Selection of the optimal pipeline diameter
Determining the optimal pipeline diameter is a complex production problem, the solution of which depends on a set of various interrelated conditions (technical and economic, characteristics of the working environment and pipeline material, technological parameters, etc.). For example, an increase in the speed of the pumped flow leads to a decrease in the diameter of the pipe that provides the media flow rate specified by the process conditions, which entails a reduction in material costs, cheaper installation and repair of the pipeline, etc. On the other hand, an increase in flow rate leads to a loss of pressure, which requires additional energy and financial costs for pumping a given volume of media.
The value of the optimal pipeline diameter is calculated using the transformed flow continuity equation, taking into account the given media flow:
In hydraulic calculations, the flow rate of the pumped liquid is most often specified by the conditions of the problem. The flow rate of the pumped medium is determined based on the properties of the given medium and the corresponding reference data (see table).
The transformed flow continuity equation for calculating the working diameter of the pipe has the form:
Formulas for calculations and reference data
Calculation of the heat load for heating involves determining heat losses (Tp) and boiler power (Mk). The latter is calculated by the formula:
- Mk – thermal performance of the heating system, kW;
- Тп – heat losses of the house;
- 1.2 – safety factor (20%).
A twenty percent safety factor allows you to take into account a possible drop in pressure in the gas pipeline during the cold season and unexpected heat losses (for example, a broken window, poor-quality thermal insulation of entrance doors or unprecedented frosts). It allows you to insure yourself against a number of troubles, and also makes it possible to widely regulate the temperature regime.
As can be seen from this formula, the boiler power directly depends on heat loss. They are not evenly distributed throughout the house: the external walls account for about 40% of the total value, the windows - 20%, the floor - 10%, the roof - 10%. The remaining 20% evaporates through doors and ventilation.
Poorly insulated walls and floors, cold attics, conventional glazing on windows - all this leads to large heat losses, and, consequently, to an increase in the load on the heating system
When building a house, it is important to pay attention to all elements, because even poorly thought-out ventilation in the house will release heat to the street. The materials from which a house is built have a direct impact on the amount of heat lost. Therefore, when making calculations, you need to analyze what the walls, the floor, and everything else are made of.
Therefore, when making calculations, you need to analyze what the walls, the floor, and everything else are made of.
The materials from which a house is built have a direct impact on the amount of heat lost. Therefore, when making calculations, you need to analyze what the walls, the floor, and everything else are made of.
In calculations, to take into account the influence of each of these factors, the corresponding coefficients are used:
- K1 – window type;
- K2 – wall insulation;
- K3 – ratio of floor area to windows;
- K4 – minimum temperature outside;
- K5 – the number of external walls of the house;
- K6 – number of storeys;
- K7 – room height.
For windows, the heat loss coefficient is:
- conventional glazing – 1.27;
- double-glazed window – 1;
- three-chamber double-glazed window - 0.85.
Naturally, the last option will keep the house warm much better than the previous two.
Properly performed wall insulation is the key not only to the long life of the house, but also to a comfortable temperature in the rooms. Depending on the material, the value of the coefficient also changes:
- concrete panels, blocks – 1.25-1.5;
- logs, beams – 1.25;
- brick (1.5 bricks) – 1.5;
- brick (2.5 bricks) – 1.1;
- foam concrete with increased thermal insulation – 1.
The larger the window area relative to the floor, the more heat the house loses:
Pressure loss calculation
The operating pressure in the pipeline is the higher excess pressure at which the specified mode of the technological process is ensured. The minimum and maximum pressure values, as well as the physical and chemical properties of the working medium, are the determining parameters when calculating the distance between the pumps pumping the media and the production capacity.
Calculation of losses due to pressure drop in the pipeline is carried out according to the equation:
general information
Basic moments
The absence of a circulation pump and generally moving elements and a closed circuit in which the amount of suspensions and mineral salts is finite makes the service life of this type of heating system very long. When using galvanized or polymer pipes and bimetallic radiators - at least half a century. Natural circulation of heating means a fairly small pressure drop. Pipes and heating devices inevitably provide a certain resistance to the movement of the coolant. That is why the recommended radius of the heating system we are interested in is estimated at approximately 30 meters. Of course, this does not mean that with a radius of 32 meters the water will freeze - the border is quite arbitrary. The inertia of the system will be quite large. Several hours may pass between lighting or starting the boiler and stabilizing the temperature in all heated rooms. The reasons are clear: the boiler has to warm up the heat exchanger, and only then the water will begin to circulate, and quite slowly. All horizontal sections of pipelines are made with a mandatory slope in the direction of water movement. It will ensure the free movement of cooling water by gravity with minimal resistance
What is equally important is that in this case all air pockets will be forced out to the top point of the heating system, where an expansion tank is mounted - sealed, with an air vent, or open.
All the air will collect at the top point.
Self-regulation
Heating a house with natural circulation is a self-regulating system. The colder the house, the faster the coolant circulates. How it works?
The fact is that the circulation pressure depends on:
Differences in height between the boiler and the lower heating device. The lower the boiler is relative to the lower radiator, the faster the water will flow into it by gravity. The principle of communicating vessels, remember? This parameter is stable and unchanged during operation of the heating system.
The diagram clearly demonstrates the principle of heating operation.
As the coolant temperature drops, its density increases, and it begins to quickly displace heated water from the lower part of the circuit.
Circulation speed
In addition to the pressure, the circulation rate of the coolant will be determined by a number of other factors.
- Diameter of distribution pipes. The smaller the internal cross-section of the pipe, the greater resistance it will provide to the movement of liquid in it. That is why, for distribution in the case of natural circulation, pipes with a deliberately oversized diameter are taken - DN32 - DN40.
- Pipe material. Steel (especially if damaged by corrosion and covered with deposits) has several times more resistance to flow than, for example, a polypropylene pipe with the same cross-section.
- Number and radius of turns. Therefore, if possible, it is better to make the main wiring as straight as possible.
- The presence, quantity and type of shut-off valves, various retaining washers and pipe diameter transitions.
Every valve, every bend causes a drop in pressure.
It is precisely because of the abundance of variables that an accurate calculation of a heating system with natural circulation is performed extremely rarely and gives very approximate results. In practice, it is enough to use the recommendations already given.
Selecting the appropriate pipe diameter for heating
It is almost impossible to accurately calculate the pipeline cross-section. For these purposes, several methods are used, with approximately the same final result. As you know, the main task of the system is to deliver the required amount of heat to the batteries in order to achieve maximum uniform heating of the heating device.
In forced circuits, a pipeline, coolant and circulation pump are used for these purposes. Using this set of devices, it is necessary to supply the required portion of the coolant in a fixed time. There are two ways to achieve this task - using smaller diameter pipes in combination with a higher speed of water movement, or using a system with a larger cross-section in which the traffic intensity will be lower.
Reasons for the popularity of the first option:
- Lower price for thinner pipes.
- Great ease of installation.
- In open areas such systems are less noticeable. If they are placed on the floor or walls, smaller installation spaces are required.
- Narrow pipes contain less liquid. This leads to a decrease in system inertia and fuel savings.
Thanks to a set of standard diameters and a fixed amount of heat transported through them, there is no need to carry out the same type of calculations. For these purposes, special tables have been compiled: they allow, having on hand data on the required amount of heat, water supply speed and operating temperature of the heating circuit, to calculate the required dimensions. To determine what pipe diameters there are for heating, you need to find the necessary table.
To calculate the diameter of heating pipes, the following formula is used: D = √354x(0.86x Q/∆t)/V, where D is the desired pipeline diameter (mm), ∆t° is the temperature delta (the difference between supply and return), Q is load for a given section of the system, kW - a certain amount of heat required to heat the room, V - coolant velocity (m/s).
Autonomous systems usually have a coolant movement speed of 0.2 - 1.5 m/s. As practical experience shows, the most optimal speed in such cases is 0.3 m/s - 0.7 m/s. When this indicator decreases, there is a real threat of air jams; when it increases, the coolant begins to make a lot of noise when moving.
There are tables to select the optimal value. They contain data for pipes made of different materials - metal, polypropylene, metal-plastic, copper. When determining the diameter of heating pipes, as a rule, the emphasis was placed on standard operating conditions with high and medium temperatures. Some examples will help you understand the essence of the procedure.
Calculation by stages
Have you determined the initial data: drawn a diagram of the heating system, decided on the type, and timely calculated the amount of thermal power for all rooms? Then proceed further. Usually the calculation starts from the most remote room.
The volumetric flow rate of liquid is calculated using the formula:
G = 0.86*Q / 20 where: G – volumetric coolant flow rate, kg/h; Q – estimated amount of heat, W; 20 – temperature difference in supply and return. For calculations it is equal to 20 °C.
Using the above formula, the mass of the liquid is determined, but hot water is characterized at 80 °C by density p = 971.6 kg/m³. Therefore, the volume flow Vo is calculated by the formula:
Vo = G/r
If you know the volume and speed of movement, it is not difficult to calculate the cross-sectional area:
S = Vo / (3600 x Vt) where: S – cross-sectional area; Vo – flow rate (volume) of the coolant; Vt – selected fluid flow rate.
And at the end, the diameter is calculated:
D = square root of 4S/3.14.
Once you have calculated the diameter for the back room, calculating the size of the pipeline for the next room is not difficult. However, it is worth remembering that the total amount of heat for two rooms, etc. must be passed through this room. The calculation as a whole is not difficult, but for those who have not previously engaged in such calculations, it is quite cumbersome.
Therefore, in order to facilitate the process itself, there are tables that give the answer and solve the problem - how to determine the diameter of the heating pipe. From the tables it is clearly clear: the diameter of heating pipes with natural circulation needs to be large, since the flow speed is 0.3 m/s. It is worth choosing pipes according to the nearest larger diameter, taking into account the discrepancy in the logic of marking pipes made of different materials:
- Water and gas pipes made of steel - the internal diameter is specified.
- Electrically welded steel products - outer diameter.
- Polyethylene, metal-plastic, low-density polyethylene, polypropylene pipes for heating - outer diameter.
Diameters of copper and steel pipes for heating:
Pipe diameter table
When you need to dot all the i’s
But a completely reasonable question arises. “How can you count something that is invisible and capable of disappearing instantly, literally out the window?” There is no need to despair of this struggle with the air; it turns out that there are quite clear mathematical calculations of the calories received for heating.
Moreover, all these calculations are hidden in official documents of state utility organizations. As usual in these institutions, there are several such documents, but the main one is the so-called “Rules for accounting of thermal energy and coolant”. It is he who will help solve the question - how to calculate Gcal for heating.
The actual problem can be solved quite simply and no calculations will be needed if you have a meter not just for water, but for hot water. The readings of such a meter are already “filled” with data on the heat received. When taking readings, you multiply it by the cost tariff and get the result.
Basic formula
The situation becomes more complicated if you do not have such a counter. Then you will have to be guided by the following formula:
- Q is the amount of thermal energy;
- V – volume of hot water consumption in cubic meters or tons;
- T1 - hot water temperature in degrees Celsius. More precisely, use temperature in the formula, but reduced to the corresponding pressure, the so-called “enthalgy”. But in the absence of a better, appropriate sensor, we simply use the temperature, which is close to enthalgy. Professional heat metering units are capable of calculating enthalgy. Often this temperature is not available for measurement, so they are guided by the constant “from the Housing Office”, which can be different, but is usually 60-65 degrees;
- T2 is the cold water temperature in degrees Celsius. This temperature is taken from the cold water pipe of the heating system. Consumers, as a rule, do not have access to this pipeline, so it is customary to take constant recommended values depending on the heating season. in season - 5 degrees; off-season – 15;
- The “1000” coefficient allows you to get rid of 10-digit numbers and get data in gigacalories (not just calories).
As follows from the formula, it is more convenient to use a closed heating system, into which the required volume of water is once poured and in the future there is no further supply. But in this case, you are prohibited from using hot water from the system.
The latest developments in the field of radiators, to some extent, may allow you to keep warm, but the desire to count everything will still not disappear.
The use of a closed system forces us to slightly improve the given formula, which already takes the form:
Q = ( (V1 * ( T1 – T ) ) – ( V2 * ( T2 – T ) ) ) / 1000
- V1 is the coolant flow rate in the supply pipeline, regardless of whether the coolant is water or steam;
- V2 - coolant flow in the return pipeline;
- T1 is the temperature of the coolant at the inlet, in the supply pipeline;
- T2 is the temperature of the coolant at the outlet, in the return pipeline;
- T - cold water temperature.
Thus, the formula consists of the difference of two factors - the first gives the value of the heat received in calories, the second - the value of the heat output.
Helpful advice! As you can see, there is not a lot of mathematics, but you still have to carry out calculations. Of course, you can immediately rush to your calculator on your mobile phone. But he advises you to create simple formulas in one of the most famous computer office programs - the so-called Microsoft Excel spreadsheet processor. included in the Microsoft Office package. In Excel, you can not only quickly calculate everything, but also “play” with the source data and simulate various situations. Moreover, Excel will help you build graphs of heat receipt and consumption, and this is an “unkillable” map for a future possible conversation with government agencies.
Video description
This video talks about the pressure drop when a pipe narrows:
Hydraulic slope refers to the angle at which the pipe is located, satisfying a certain condition. If the sewer pipe is located in this way, then under the influence of gravity its contents should drain in such a way that self-cleaning occurs. This value is determined on the basis of special tables.
When calculating the hydraulic radius, they are based on the actual diameter of the pipe and the degree of its filling with contents. So, for example, for a completely filled product these two radii will be equal, but with partial filling the hydraulic radius will be smaller. It is calculated based on appropriate formulas or tables.
Properly designed and installed sewer pipes will ensure good drainage and eliminate the risk that they may become clogged.
When calculating the throughput of a gas system pipe, it must be taken into account that a gaseous, not a liquid, substance spreads through them. One of the important differences is the high compression ratio.
The pipe diameter must be selected correctly Source otoplenie.site
When transporting gas, the pipe capacity depending on the diameter is calculated using the following formula.
Q = DN * 0.67 * p
This formula uses the following notation:
- Q represents the maximum capacity of the gas pipe;
- DN is equal to the nominal diameter of the gas pipe;
- p is a value equal to the operating gas pressure, which must be increased by 10%.
The throughput of a gas pipeline can be calculated using the formula given here or using a table compiled on its basis. This formula is used to calculate the parameters of pipes in residential buildings and apartments.
For calculations in industry, a different formula is used.
Q = DN2 * 196.386 * (p/z) * T
The following notations were used here:
- Q is the pipeline capacity;
- DN2 indicates the diameter of the pipe;
- z – compression ratio;
- p – pressure in the pipeline;
- T – gas temperature.
Heating pipes Source woodmaster-shop.ru
If the temperature increases, this will lead to increased pressure on the pipe walls. Therefore, when using gas, it is necessary to take into account climatic conditions, which significantly affect the permeability of the pipeline. Using a diameter that is too small increases the risk of increased gas pressure and pipe damage.